User Tools
Sidebar
This is an old revision of the document!
We are Culinary Services.
Who ordered the extra side of 44Ti?
GROUP MEMBERS
Alex Long (parameters)
MacKenzie Warren (code)
Nathan Parzuchowski (analysis)
TOPIC
Sensitivity studied of 44Ti production in in core-collapse supernova environments.
Scientific Background
There are many uncertainties in our understanding of core-collapse supernovae, including the explosion mechanism and nucleosynthesis. One way to gain insight into these phenomena is to study the nucleosynthesis of radioactive isotopes in the shock-heated material. These isotopes, such as 44Ti and 56Ni, determine the features of the supernova light curve. Observations of supernova remnants can be used to put bounds on the production of these isotopes.
 Figure: Observation of Cassiopeia A. Green shows 44Ti distribution, blue is 28Si, and the red shows the Fe distribution. (From Grefenstette et al 2014)
Figure: Observation of Cassiopeia A. Green shows 44Ti distribution, blue is 28Si, and the red shows the Fe distribution. (From Grefenstette et al 2014)
Using simulations, we can use these observations to gain insight into the supernova environment. By matching observed abundances, we can gain insight into the environment in which this nucleosynthesis must have taken place and in turn, the details of the explosion mechanism. However, most core-collapse supernova simulations do not include sufficiently large reaction networks to simulate this nucleosynthesis.
If the shock heating is sufficient, the material will be in Nuclear Statistical Equilibrium (NSE). The isotopic abundances will be set by the thermodynamic environment (i.e. temperature and density).
Simulations
We have chosen to do a parameter space study in peak temperature, density, and electron fraction, tarting with a set parameter space of peak temperatures [T9 = 4 - 7] and densities [$\rho$ = 105 - 107 g/cm3] for three values of the electron fraction [Ye = 0.45, 0.50, 0.55]. This parameter space roughly corresponds with the shock heated region in simulations of Cassiopeia A-like supernovae (Young & Fryer 2007).
We use analytic adiabatic freeze-out trajectories (Hoyle et al. 1964; Fowler & Hoyle 1964) which satisfy the differential equations:
\begin{equation} \frac{dT}{dt} = \frac{-T}{3\tau} \hspace{1cm} \frac{d\rho}{dt} = -\frac{\rho}{\tau} \end{equation}
where $\tau$ is the hydrodynamic timescale. This leads to temperature and density trajectories:
\begin{equation} T(t) = T_0 exp(-t/3\tau) \hspace{1cm} \rho (t) = \rho_0 exp(-t/\tau) \end{equation} where $T_0$ and $\rho_0$ are the peak temperature and density in the supernova.
We used the XNet reaction network code. Our code included 447 isotopes ranging from hydrogen through germanium. We took the reaction rates from the JINA Reaclib database. We set the threshold temperature for NSE to be 5 GK.
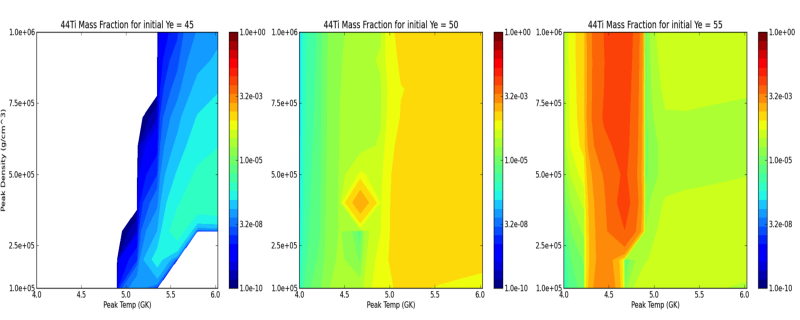
Finally we looked at the mass fraction of several isotopes. In particular, $^{4}$He, $^{28}$Si, $^{44}$Ti, and $^{56}$Ni. We then compare our results to that of Magkotsios et al with in our parameter space.
Results
$^{44}Ti
REFERENCES
Trends in 44Ti and 56Ni from Core-Collapse Supernovae (Magkotsios et al 2010)
Nuclear Reactions Governing the Nucleosynthesis of 44Ti (The et al 1998)
X-ray and gamma-ray studies of Cas A (Vink 2004)
Uncertainties in Supernova Yields I One-dimensional Explosions (Young & Fryer 2007)
Asymmetries in core-collapse supernovae from maps of radioactive 44Ti in Cassiopeia A (Grefenstette et al 2014)




